Math Symbols and Their Names

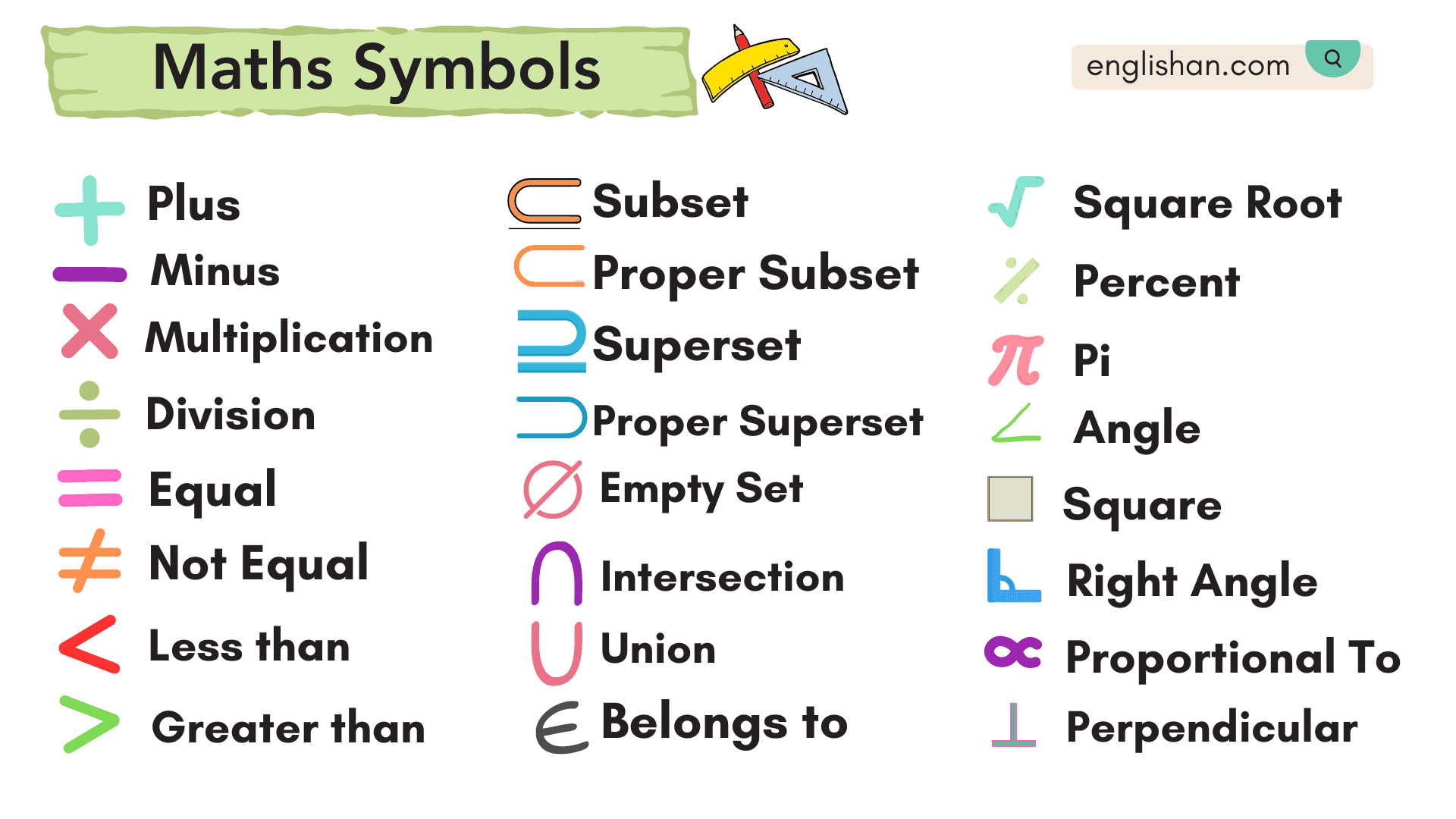
Contents

9 Minutes Read

Math symbols are essential in expressing mathematical ideas and solving equations. Each symbol, from addition and subtraction to multiplication and division, plays a unique role in helping us understand complex concepts. Learning math symbols and their names is crucial for anyone wanting to improve their math skills. These symbols are not just used in classrooms but also in our daily lives.

Basic Math Symbols

Addition (+) and Subtraction (−)

The addition symbol (+) is one of the most fundamental math symbols. It signifies the process of combining two or more numbers to get a total. For example, in the equation $2 + 3 = 5$ , the plus sign adds 2 and 3 together.

The subtraction symbol (−) represents the operation of removing one quantity from another. For instance, $5 - 2 = 3$ means subtracting 2 from 5.

Multiplication (×) and Division (÷)

Among the core math symbols, the *multiplication symbols (× or ) indicate that you multiply numbers together. For example, $4 \times 3 = 12$ .

The division symbols (÷ or /) are used to divide one number by another. For instance, $8 \div 2 = 4$ .

Equals (=) and Not Equal (≠)

The equals symbol (=) is a basic yet powerful math symbol showing that the values on either side of it are the same. For example, $2 + 3 = 5$ means that the sum of 2 and 3 is equal to 5.

The not equal symbol (≠) indicates that two values are not the same. For example, $3 \neq = 4$ .

Inequality Symbols (<, >, ≤, ≥)

Less than (<): This math symbol indicates that one number is smaller than another, e.g., $3 < 5$ .
Greater than (>): Indicates that one number is larger than another, e.g., $5 > 3$ .
Less than or equal to (≤): Means a number is either less than or equal to another, e.g., $x \leq 5$ .
Greater than or equal to (≥): Means a number is either greater than or equal to another, e.g., $x \geq 5$ .

Percent (%)

The percent symbol (%) is one of the most commonly used math symbols and indicates a part per hundred. For example, 50% means 50 out of 100.

Approximate (≈)

The approximately equal symbol (≈) is another crucial math symbol, showing that two values are close but not exactly equal. For example, $π \approx 3.14$ .

Degree (°)

The degree symbol (°) is used to denote angles or temperatures. For example, $90°$ indicates a right angle.

Angle (∠)

The angle symbol (∠) represents the measure of an angle. For instance, $∠ A BC = 45°$ , a frequently encountered math symbol in geometry.

Parallel (∥) and Perpendicular (⊥)

Parallel (∥): This math symbol indicates that two lines are parallel, e.g., $A B ∥ C D$ .
Perpendicular (⊥): Indicates that two lines intersect at a right angle, e.g., $A B ⊥ C D$ .

Absolute Value (| |)

The absolute value symbol (| |) represents the distance of a number from zero on the number line. For instance, $∣ - 3∣ = 3$ .

Advanced Math Symbols

Pi (π)

The pi symbol (π) is one of the most recognized math symbols, representing the mathematical constant approximately equal to 3.14159. It’s the ratio of the circumference of a circle to its diameter.

Infinity (∞)

The infinity symbol (∞) signifies a value that grows without bounds. It’s often used in limits, calculus, and set theory, making it a crucial math symbol.

Integral (∫)

The integral symbol (∫) represents the process of integration in calculus. It is used to calculate areas under curves or the accumulation of quantities. Multiple integrals include $∬\iint$ , $∭\iiint$ , and $∮\oint$ for line integrals.

Summation (Σ)

The summation symbol (Σ) is used to sum up a series of terms. For example, $Σi=1ni\Sigma_{i=1}^{n} i$ adds all integers from 1 to $n$ , making it an essential math symbol in algebra and calculus.

Delta (Δ)

The delta symbol (Δ) is often used to denote a change or difference in a variable. For instance, $Δx\Delta x$ represents a change in the variable $x$ , a key concept in advanced mathematics.

Square Root (√)

The square root symbol (√) indicates the principal square root of a number. For example, $\sqrt9 = 3$ , because 3 squared equals 9. This math symbol is fundamental in algebra.

Proportional (∝)

The proportional symbol (∝) indicates that two quantities are proportional. For example, $y \propto x$ means $y$ is directly proportional to $x$ , a concept widely used in physics and mathematics.

Similarity (∼)

The similarity symbol (∼) denotes that two figures are similar in geometry, an important math symbol in geometric proofs.

Nabla (∇)

The nabla symbol (∇), also known as the del operator, is used in vector calculus to denote gradients, divergences, and curls.

Greek Letters in Math

Alpha (α), Beta (β), Gamma (γ)

Greek letters are widely used in mathematics to represent various constants, variables, and functions. For instance:

Alpha (α): Often used to represent angles, a frequent math symbol in trigonometry.
Beta (β): Frequently appears in statistics.
Gamma (γ): Used in complex analysis and other fields.

Theta (θ)

The theta symbol (θ) is commonly used to represent angles in trigonometry, one of the most recognized math symbols.

Lambda (λ)

The lambda symbol (λ) is often used in calculus, particularly in relation to eigenvalues in linear algebra, making it a vital math symbol in advanced studies.

Set Theory Math Symbols

Union (∪)

The union symbol (∪) represents the union of two sets, meaning all elements that are in either set.

Intersection (∩)

The intersection symbol (∩) indicates the common elements between sets.

Difference (∖)

The difference symbol (∖) represents the difference between two sets, showing the elements in one set that are not in the other.

Subset (⊆) and Superset (⊇)

Subset (⊆): Indicates that all elements of one set are contained within another. $A \subseteq B$ means every element of set $A$ is also in set $B$ .
Superset (⊇): The reverse of a subset; $A \supseteq B$ means that set $A$ contains all elements of set $B$ .
Proper Subset (⊂): Indicates that set $A$ is a subset of $B$ but not equal to $B$ , i.e., $A \subset B$ .
Proper Superset (⊃): Indicates that set $A$ contains all elements of set $B$ but is not equal to $B$ , i.e., $A \supset B$ .

Element of (∈) and Not Element of (∉)

The element of symbol (∈) denotes that an item belongs to a set. For instance, $3 \in A$ means 3 is an element of set $A$ .

The not element of symbol (∉) indicates that an item does not belong to a set. For example, $3 \in / B$ .

Empty Set (∅)

The empty set symbol (∅) represents a set with no elements. It is also denoted by ${}\{\}$ , one of the fundamental math symbols in set theory.

Calculus Math Symbols

Derivative (d/dx)

The derivative symbol (d/dx) signifies the derivative of a function with respect to $x$ . For example, $d/dx(x^2) = 2x$ . This math symbol is key in differential calculus.

Partial Derivative (∂)

The partial derivative symbol (∂) is used to denote the derivative of a multivariable function with respect to one variable while keeping others constant.

Limit (lim)

The limit symbol (lim) represents the value that a function approaches as the input approaches some value. For example, $lim⁡x→0sin⁡(x)x=1\lim_{x \to 0} \frac{\sin(x)}{x} = 1$ . This math symbol is crucial in calculus.

Gradient (∇)

The gradient symbol (∇), also known as nabla, is used to denote the gradient of a scalar field in vector calculus.

Exponent ( ^ )

The exponent symbol ( ^ ) denotes raising a number to the power of another. For example, $2^3 = 8$ .

Product (∏)

The product symbol (∏) represents the product of a sequence of terms. For example, $∏i=1ni\prod_{i=1}^{n} i$ is the product of all integers from 1 to $n$ .

Summation (Σ)

The summation symbol (Σ) is used to sum up a series of terms. For example, $Σi=1ni\Sigma_{i=1}^{n} i$ adds all integers from 1 to $n$ .

Logical Symbols in Math

And (∧)

The and symbol (∧) is used in logic to indicate that both statements are true. For example, $P \land Q$ is true if both $P$ and $Q$ are true.

Or (∨)

The or symbol (∨) indicates that at least one of the statements is true. $P \lor Q$ is true if either $P$ or $Q$ (or both) are true.

Not (¬)

The not symbol (¬) is used to negate a statement. For instance, if $P$ is true, then $\neg P$ is false.

Implies (⇒)

The implies symbol (⇒) shows that one statement implies another. For example, $P \Rightarrow Q$ means if $P$ is true, then $Q$ must also be true.

Biconditional (↔)

The biconditional symbol (↔) indicates that two statements are both true or both false. For example, $P \leftrightarrow Q$ means $P$ is true if and only if $Q$ is true.

Therefore (∴) and Because (∵)

Therefore (∴): Used to conclude an argument or proof, indicating that what follows logically results from what preceded.
Because (∵): Used to provide a reason or explanation for a preceding statement.

Miscellaneous Symbols

Factorial (!)

The factorial symbol (!) denotes the product of all positive integers up to a certain number. For example, $5! = 5 \times 4 \times 3 \times 2 \times 1 = 120$ .

Circle (○)

The circle symbol (○) is often used in geometry to denote a circle.

Triangle (△) and Square (□)

Triangle (△): Represents a triangle in geometry.
Square (□): Represents a square in geometry.

Homothetic (≺, ≻)

The homothetic symbols (≺, ≻) are used in geometry to denote a relation between homothetic figures.

Isomorphism (≡)

The isomorphism symbol (≡) represents that two structures are isomorphic, meaning they are structurally identical.

Math Symbols Infographics

Conclusion

Understanding math symbols is crucial for solving mathematical problems and communicating ideas effectively. Whether you’re dealing with basic arithmetic or advanced calculus, familiarizing yourself with these symbols will enhance your mathematical fluency. By categorizing them appropriately and understanding their meanings, you can navigate the world of mathematics with greater confidence and precision.

FAQs:

1. What are the basic math symbols?

Here are the basic math symbols:
1. +for addition (2 + 3 = 5)
2. -for subtraction (5 – 2 = 3)
3. × for multiplication (3 × 4 = 12)
4. ÷ for division (12 ÷ 4 = 3)
5. = means equal (2 + 2 = 4)
6. < means less than (3 < 5)
7. >means greater than (7 > 2)
These symbols are simple and used in everyday math!

2. What is ∈ called in math?

In math, ∈ is called “element of”.
It means that something belongs to a set. For example:
x ∈ A means “x is an element of set A.”
If A = {1, 2, 3}, then 2 ∈ A (because 2 is in the set).
It’s a common symbol in set theory.

3. What is ∑ called?

In math, ∑ is called “sigma” or “summation symbol”.
It is used to represent the sum of a series of numbers. For example:
∑ (i = 1 to 4) i means 1 + 2 + 3 + 4 = 10.
It’s commonly used in algebra and calculus for adding terms in a sequence.

4. What is := in math?

In math, := means “is defined as” or “equals by definition.”
It is used to define a new variable, function, or expression. For example:
f(x) := x² + 2 means the function f(x) is defined as x² + 2.
This symbol helps clarify definitions.

5. What is ∑ vs ∏?

The symbols ∑ and ∏ are used for different purposes in math:
∑ (Sigma) – Represents summation (adding numbers).
Example: ∑ (i = 1 to 4) i = 1 + 2 + 3 + 4 = 10
∏ (Pi) – Represents product (multiplying numbers).
Example: ∏ (i = 1 to 4) i = 1 × 2 × 3 × 4 = 24
Key Difference:
∑ adds numbers together.
∏ multiplies numbers together.